| << Chapter < Page | Chapter >> Page > |
A polynomial with two terms is called a binomial. We have already learned to multiply binomials and to raise binomials to powers, but raising a binomial to a high power can be tedious and time-consuming. In this section, we will discuss a shortcut that will allow us to find without multiplying the binomial by itself times.
In Counting Principles , we studied combinations . In the shortcut to finding we will need to use combinations to find the coefficients that will appear in the expansion of the binomial. In this case, we use the notation instead of but it can be calculated in the same way. So
The combination is called a binomial coefficient . An example of a binomial coefficient is
If and are integers greater than or equal to 0 with then the binomial coefficient is
Is a binomial coefficient always a whole number?
Yes. Just as the number of combinations must always be a whole number, a binomial coefficient will always be a whole number.
Find each binomial coefficient.
Use the formula to calculate each binomial coefficient. You can also use the function on your calculator.
Find each binomial coefficient.
When we expand by multiplying, the result is called a binomial expansion , and it includes binomial coefficients. If we wanted to expand we might multiply by itself fifty-two times. This could take hours! If we examine some simple binomial expansions, we can find patterns that will lead us to a shortcut for finding more complicated binomial expansions.
First, let’s examine the exponents. With each successive term, the exponent for decreases and the exponent for increases. The sum of the two exponents is for each term.
Next, let’s examine the coefficients. Notice that the coefficients increase and then decrease in a symmetrical pattern. The coefficients follow a pattern:
These patterns lead us to the Binomial Theorem , which can be used to expand any binomial.
Another way to see the coefficients is to examine the expansion of a binomial in general form, to successive powers 1, 2, 3, and 4.
Can you guess the next expansion for the binomial
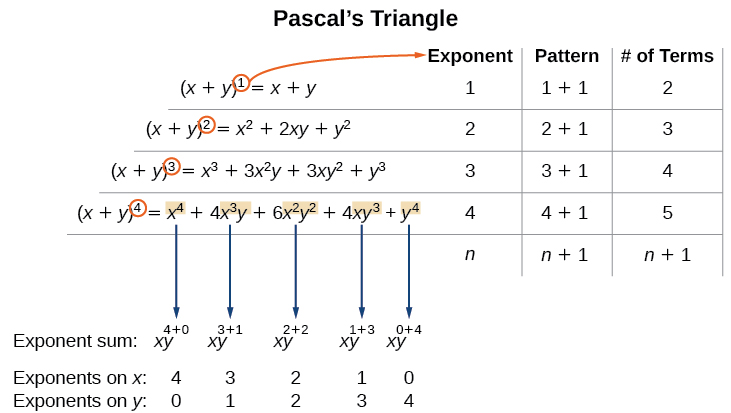
See [link] , which illustrates the following:
To determine the expansion on we see thus, there will be 5+1 = 6 terms. Each term has a combined degree of 5. In descending order for powers of the pattern is as follows:

Notification Switch
Would you like to follow the 'Algebra and trigonometry' conversation and receive update notifications?