| << Chapter < Page | Chapter >> Page > |
Quarks have been mentioned at various points in this text as fundamental building blocks and members of the exclusive club of truly elementary particles. Note that an elementary or fundamental particle has no substructure (it is not made of other particles) and has no finite size other than its wavelength. This does not mean that fundamental particles are stable—some decay, while others do not. Keep in mind that all leptons seem to be fundamental, whereas no hadrons are fundamental. There is strong evidence that quarks are the fundamental building blocks of hadrons as seen in [link] . Quarks are the second group of fundamental particles (leptons are the first). The third and perhaps final group of fundamental particles is the carrier particles for the four basic forces. Leptons, quarks, and carrier particles may be all there is. In this module we will discuss the quark substructure of hadrons and its relationship to forces as well as indicate some remaining questions and problems.
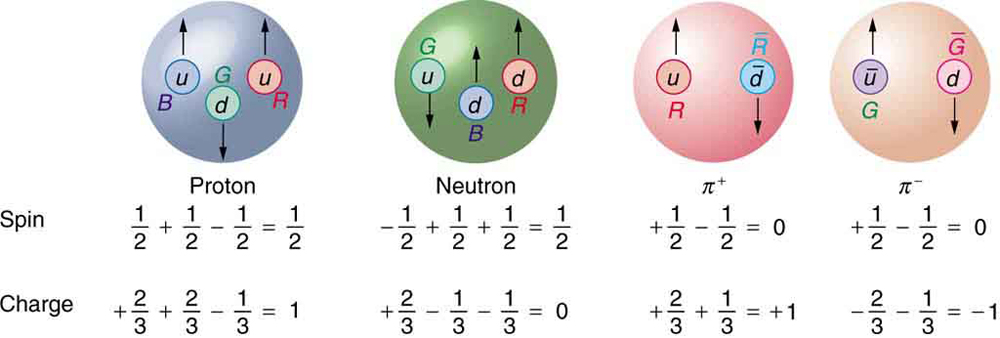
Quarks were first proposed independently by American physicists Murray Gell-Mann and George Zweig in 1963. Their quaint name was taken by Gell-Mann from a James Joyce novel—Gell-Mann was also largely responsible for the concept and name of strangeness. (Whimsical names are common in particle physics, reflecting the personalities of modern physicists.) Originally, three quark types—or flavors —were proposed to account for the then-known mesons and baryons. These quark flavors are named up ( u ), down ( d ), and strange ( s ). All quarks have half-integral spin and are thus fermions. All mesons have integral spin while all baryons have half-integral spin. Therefore, mesons should be made up of an even number of quarks while baryons need to be made up of an odd number of quarks. [link] shows the quark substructure of the proton, neutron, and two pions. The most radical proposal by Gell-Mann and Zweig is the fractional charges of quarks, which are and , whereas all directly observed particles have charges that are integral multiples of . Note that the fractional value of the quark does not violate the fact that the e is the smallest unit of charge that is observed, because a free quark cannot exist. [link] lists characteristics of the six quark flavors that are now thought to exist. Discoveries made since 1963 have required extra quark flavors, which are divided into three families quite analogous to leptons.
To understand how these quark substructures work, let us specifically examine the proton, neutron, and the two pions pictured in [link] before moving on to more general considerations. First, the proton p is composed of the three quarks uud , so that its total charge is , as expected. With the spins aligned as in the figure, the proton’s intrinsic spin is , also as expected. Note that the spins of the up quarks are aligned, so that they would be in the same state except that they have different colors (another quantum number to be elaborated upon a little later). Quarks obey the Pauli exclusion principle. Similar comments apply to the neutron n , which is composed of the three quarks udd . Note also that the neutron is made of charges that add to zero but move internally, producing its well-known magnetic moment. When the neutron decays, it does so by changing the flavor of one of its quarks. Writing neutron decay in terms of quarks,

Notification Switch
Would you like to follow the 'College physics' conversation and receive update notifications?