10.6 Collisions of extended bodies in two dimensions

Learning objectives

By the end of this section, you will be able to:

• Observe collisions of extended bodies in two dimensions.
• Examine collisions at the point of percussion.

The information presented in this section supports the following AP® learning objectives and science practices:

• 3.F.3.1 The student is able to predict the behavior of rotational collision situations by the same processes that are used to analyze linear collision situations using an analogy between impulse and change of linear momentum and angular impulse and change of angular momentum. (S.P. 6.4, 7.2)
• 3.F.3.2 In an unfamiliar context or using representations beyond equations, the student is able to justify the selection of a mathematical routine to solve for the change in angular momentum of an object caused by torques exerted on the object. (S.P. 2.1)
• 3.F.3.3 The student is able to plan data collection and analysis strategies designed to test the relationship between torques exerted on an object and the change in angular momentum of that object. (S.P. 4.1, 4.2,, 5.1, 5.3)
• 4.D.2.1 The student is able to describe a model of a rotational system and use that model to analyze a situation in which angular momentum changes due to interaction with other objects or systems. (S.P. 1.2, 1.4)
• 4.D.2.2 The student is able to plan a data collection and analysis strategy to determine the change in angular momentum of a system and relate it to interactions with other objects and systems. (S.P. 2.2)

Bowling pins are sent flying and spinning when hit by a bowling ball—angular momentum as well as linear momentum and energy have been imparted to the pins. (See [link] ). Many collisions involve angular momentum. Cars, for example, may spin and collide on ice or a wet surface. Baseball pitchers throw curves by putting spin on the baseball. A tennis player can put a lot of top spin on the tennis ball which causes it to dive down onto the court once it crosses the net. We now take a brief look at what happens when objects that can rotate collide.

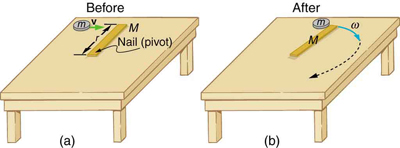
Consider the relatively simple collision shown in [link] , in which a disk strikes and adheres to an initially motionless stick nailed at one end to a frictionless surface. After the collision, the two rotate about the nail. There is an unbalanced external force on the system at the nail. This force exerts no torque because its lever arm $r$ is zero. Angular momentum is therefore conserved in the collision. Kinetic energy is not conserved, because the collision is inelastic. It is possible that momentum is not conserved either because the force at the nail may have a component in the direction of the disk's initial velocity. Let us examine a case of rotation in a collision in [link] .