| << Chapter < Page | Chapter >> Page > |
Kinetic energy is energy of motion. Classically, kinetic energy has the familiar expression . The relativistic expression for kinetic energy is obtained from the work-energy theorem. This theorem states that the net work on a system goes into kinetic energy. If our system starts from rest, then the work-energy theorem is
Relativistically, at rest we have rest energy . The work increases this to the total energy . Thus,
Relativistically, we have .
Relativistic kinetic energy is
When motionless, we have and
so that at rest, as expected. But the expression for relativistic kinetic energy (such as total energy and rest energy) does not look much like the classical . To show that the classical expression for kinetic energy is obtained at low velocities, we note that the binomial expansion for at low velocities gives
A binomial expansion is a way of expressing an algebraic quantity as a sum of an infinite series of terms. In some cases, as in the limit of small velocity here, most terms are very small. Thus the expression derived for here is not exact, but it is a very accurate approximation. Thus, at low velocities,
Entering this into the expression for relativistic kinetic energy gives
So, in fact, relativistic kinetic energy does become the same as classical kinetic energy when .
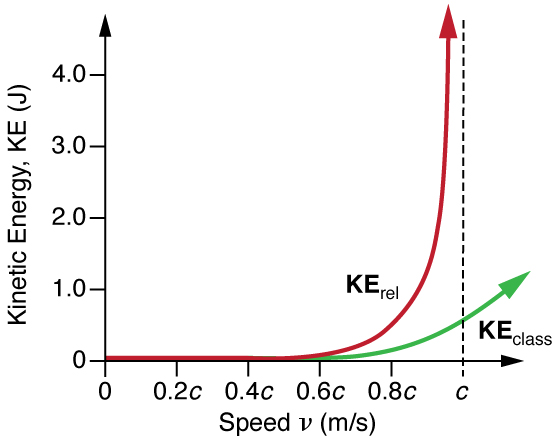
It is even more interesting to investigate what happens to kinetic energy when the velocity of an object approaches the speed of light. We know that becomes infinite as approaches , so that KE rel also becomes infinite as the velocity approaches the speed of light. (See [link] .) An infinite amount of work (and, hence, an infinite amount of energy input) is required to accelerate a mass to the speed of light.
No object with mass can attain the speed of light.
So the speed of light is the ultimate speed limit for any particle having mass. All of this is consistent with the fact that velocities less than always add to less than . Both the relativistic form for kinetic energy and the ultimate speed limit being have been confirmed in detail in numerous experiments. No matter how much energy is put into accelerating a mass, its velocity can only approach—not reach—the speed of light.
An electron has a velocity . (a) Calculate the kinetic energy in MeV of the electron. (b) Compare this with the classical value for kinetic energy at this velocity. (The mass of an electron is .)

Notification Switch
Would you like to follow the 'College physics for ap® courses' conversation and receive update notifications?