| << Chapter < Page | Chapter >> Page > |
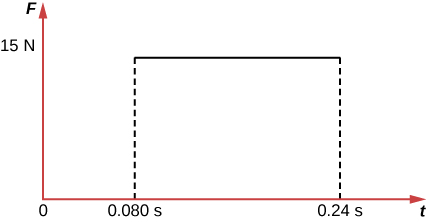
A 1.2-kg block slides across a horizontal, frictionless surface with a constant speed of 3.0 m/s before striking a fixed barrier and coming to a stop. In [link] , the force exerted by the barrier is assumed to be a constant 15 N during the 0.24-s collision. The impulse can be calculated using the area under the curve.
Note that the initial momentum of the block is:
We are assuming that the initial velocity is −3.0 m/s. We have established that the force exerted by the barrier is in the positive direction, so the initial velocity of the block must be in the negative direction. Since the final momentum of the block is zero, the impulse is equal to the change in momentum of the block.
Suppose that, instead of striking a fixed barrier, the block is instead stopped by a spring.Consider the force exerted by the spring over the time interval from the beginning of the collision until the block comes to rest.
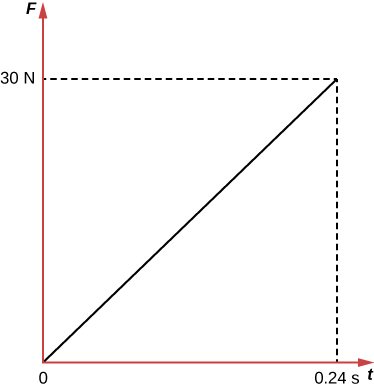
In this case, the impulse can be calculated again using the area under the curve (the area of a triangle):
Again, this is equal to the difference between the initial and final momentum of the block, so the impulse is equal to the change in momentum.
Two identical billiard balls strike a rigid wall with the same speed, and are reflected without any change of speed. The first ball strikes perpendicular to the wall. The second ball strikes the wall at an angle of from the perpendicular, and bounces off at an angle of from perpendicular to the wall.
(a) Determine the direction of the force on the wall due to each ball.
(b) Calculate the ratio of the magnitudes of impulses on the two balls by the wall.
Strategy for (a)
In order to determine the force on the wall, consider the force on the ball due to the wall using Newton’s second law and then apply Newton’s third law to determine the direction. Assume the -axis to be normal to the wall and to be positive in the initial direction of motion. Choose the -axis to be along the wall in the plane of the second ball’s motion. The momentum direction and the velocity direction are the same.
Solution for (a)
The first ball bounces directly into the wall and exerts a force on it in the direction. Therefore the wall exerts a force on the ball in the direction. The second ball continues with the same momentum component in the direction, but reverses its -component of momentum, as seen by sketching a diagram of the angles involved and keeping in mind the proportionality between velocity and momentum.
These changes mean the change in momentum for both balls is in the direction, so the force of the wall on each ball is along the direction.

Notification Switch
Would you like to follow the 'College physics for ap® courses' conversation and receive update notifications?